Do stringy electrons spin faster than light?
Luboš Motl, August 01, 2014
No. String theory’s resolution of the old paradox is a sign of the hidden cleverness of string theory.
After he read some of my essays on the electron’s spin, Tom W. Larkin asked an interesting question:
Does string theory resolve the paradox of (post-)classical physics that the electron, if imagined as a spinning ball of a very small radius, has to rotate faster than the speed of light for its spin to be \(\hbar/2\)?
One natural, fast, legitimate, but cheap reaction is to say: the electron isn’t really a rotating ball. The spin may be carried even by a point-like particle, without any violations of relativity, as QED shows, so the paradox has never been there.

Of course that a string theorist is likely to answer in this way, too. Quantum field theory is a limit of string theory so any explanation that was OK within quantum field theory may be said to be correct within string theory, too. The paradox doesn’t exist because the electron isn’t a classical ball that gets the mass from the electrostatic self-interaction energy.
However, string theory does represent the electron (and other elementary particles) as some kind of an extended object which is qualitatively analogous to the rotating ball so some version of the «superluminal spinning» paradox may be said to reemerge in string theory. Does it cause inconsistencies within string theory?
It doesn’t but the reasons are tricky and ingenious, if you allow me to lick Nature’s buttocks a little bit.
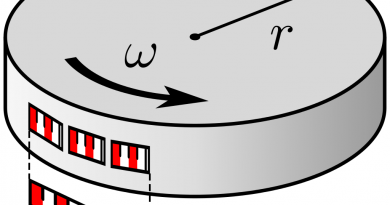
The angular momentum (I will call it «spin») of a gyroscope etc. is equal to
\[ \vec S = I\vec \omega \]
where \(\omega\) is the angular frequency and \(I\) is the moment of inertia. Up to constants of order one, the moment of inertia is equal to
\[ I \sim mr^2 \]
where \(r\) is some typical distance of the points of the object from the axis of rotation and \(m\) is the mass of the spinning object. That was some elementary classical mechanics, OK?
Now, if you assume that \(E=mc^2\) for the electron where \(m\) is the rest mass and that the full latent energy \(E\) is obtained as some electrostatic energy of the electron’s charge \(e\) interacting with itself, via (up to numerical constants of order one)
\[ E \sim \frac{e^2}{4\pi \epsilon_0 r} \]
then you may derive the typical distance \(r\) between the «pieces of the charge» of the electron i.e. the «classical electron radius» which is a few femtometers (that is \(10^{-15}\) meters).
If you know \(r\) and \(m\), you may calculate the moment of inertia \(I\sim mr^2\) as well as the angular frequency \(\omega\sim I/S\sim I/\hbar \). When you multiply this \(\omega\) by the radius \(r\) again, you get the velocity \(v\) of the points on the spinning surface of the electron. And it will be much higher than the speed of light!
I invite you to complete the steps above if you have never done so. You may evaluate all these things in the SI units, as if it were a basic school problem in mechanics. However, it’s also nice to calculate it as an adult physicist, in the Planck units. In the Planck units, the electron mass is something like \(10^{-23}\). Similarly, setting the fine-structure constant to one for a while (we will have to return to this approximation), the electrostatic formula above implies that \(E\sim 1/r\) and the classical radius is therefore about \(10^{23}\) Planck lengths.
The moment of inertia is roughly \(mr^2\) so there are two factors of \(10^{23}\) «up» and one factor down, so it is again \(10^{23}\) in Planck units. The angular frequency is \(\omega\sim 1 / I\) where \(1\) means \(\hbar\) and it is again of order \(10^{-23}\), but if you multiply it by the radius \(10^{23}\) to get the speed, you get something of order one (the speed of light).
To quickly see (without real calculations) whether the speed on the surface is greater than the speed of light, we must be a bit more careful about the fine-structure constant. The mass was OK, \(10^{-23}\), but the electron radius is \(137\) times smaller than we have said because this reduced \(r\) has to cancel the \(\alpha=1/137\) that appeared in the numerator. The moment of inertia is \(137^2\) times smaller than we said, because it’s \(mr^2\), and the angular velocity is therefore \(137^2\) times larger than we said because \(\hbar = I\omega\) has to be kept fixed. Even when multiplied by the \(137\) times smaller radius, we still get the velocity \(137\) times larger than we said.
The speed of the surface of the electron is therefore comparable to \(c/\alpha\sim 137 c\), up to numbers of order one that hopefully don’t reduce \(137\) below one. You see that the speed of the classical electron is higher than the speed of light. A bummer.
If you quickly and naively think about the changes that string theory makes to this calculation, string theory makes the problem worse because the electron in string theory is smaller. The energy of the electron comes from very stiff strings inside, not from the electrostatic energy, so the extended string hiding in the electron isn’t \(10^{-15}\) meters large but \(10^{-35}\) meters tiny or so, not far from the Planck length (the string length, about 100 or 1,000 times longer, would be a better estimate).
So the size of the electron has seemingly shrunk \(10^{20}\) times which means that the required velocity on the surface has to increase \(10^{20}\) times – hopelessly larger than the speed of light. Do the points on the string move with these excessive superluminal speeds?
The answer is, of course, No. String theory reduces the «classical radius» of the electron but it changes other things, too. Most importantly, it changes the relevant mass, too.
The trick is that the thing that is spinning isn’t as light as the electron. It’s as heavy as the Planck mass (again, more precisely, the string mass, the square root of the string tension). Why? Because the electron, like all massless and observably light particles, comes from the massless level of the string whose mass is constructed in a similar way as the massless level of the bosonic string theory which I pick as an example because of its simplicity. The massless open bosonic strings are given by
\[ \ket\gamma = \alpha_{-1}^\mu \ket 0 \]
I called the one-string state a «photon». A similar relationship holds for massless closed strings (the graviton, the dilaton, and the \(B\)-field) but there are two alpha excitations (one left-moving and one right-moving) in front of the tachyonic ground state \(\ket 0\).
If we want to see how the string pretending to be a point-like particle is spinning, we may see that it’s really the oscillator excitation \(\alpha_{-1}^\mu\) or the analogous excitations in the superstring case (that may include fermionic world sheet fields) that carries all the spin because it carries the \(\mu\) Lorentz vector index (or spinor indices, in the Green-Schwarz formalism for the superstrings). This \(\alpha\) oscillator literally corresponds to adding some relative motion to the individual points of the string, so that they move as a wave with one cycle (the subscript) around the string.
The tachyonic ground state \(\ket 0\) is not spinning. The tachyon is a scalar, after all. The squared mass of the tachyonic ground state (which is filtered out in superstring theory but may be still used as a useful starting point to construct the spectrum in the RNS superstring) is equal to \(-(D-2)/24\) times \(1/\alpha’\) for the open string – so it’s exactly \(-1/\alpha’\) for \(D=26\) – because the term
\[ \frac{1}{2} (1+2+3+4+5+\dots ) = -\frac{1}{24} \]
is contributed by each of the \(D-2\) transverse spatial dimensions. I’ve discussed this semi-heuristic explanation of the zero-point energies in string theory many times (calculations that avoid all of this heuristics exist, too, but they prove that the semi-heuristic treatment involving the sum of positive integers is at least morally right whether people like it or not).
And the oscillator \(\alpha_{-1}^\mu\) is increasing the squared mass back to the massless level, by \(+1/\alpha’\). And it’s the spinning part of the electron or other particles in string theory. So the relevant estimate for the mass of the «gyroscope» that we should use in string theory isn’t the tiny electron mass but the string mass, \(1/\sqrt{\alpha’}\) or so.
The estimate for the speed of the «stringy surface» of the electron is easy to calculate now. In the string units \(\hbar=c=\alpha’=1\), the radius is one, the spin is one, the moment of inertia is one, the angular velocity is therefore also one, and so is the speed of the surface. This estimate is compatible with the assumption that the pieces of the strings never really move faster than light although they get close – and more accurate derivations within string theory may be shown to confirm this claim in some detail.
Note that no counterpart of the fine-structure constant such as the string coupling \(g_{\rm string}\) entered in our calculation based on string units. Everything was comparable to the string scale – which may differ from the Planck scale by a power of \(g_{\rm string}\) but the string scale really simplifies the calculation more than the Planck scale. For \(g_{\rm string}\sim 1\), you don’t have to distinguish the string scale and the Planck scale.
The «string-scale» part of the electron in perturbative string theory is very heavy and therefore it’s easy for it to produce the angular momentum of order \(\hbar=1\) even with velocities that don’t breach the speed-of-light limit. And the tachyonic, negative contribution to the squared mass cancels most of the squared mass from the «positive excitation» and it makes the particle massless (or, when subleading effects are included, very light). This tachyonic part doesn’t enter the calculations of the gyroscope.
It’s very natural that string theory had to solve this puzzle – even though you could simply deny its existence – because string theory partly restored the assumptions that were used in the derivation of the nonsensical superluminal speed of spinning electron. You may see that string theory is a typical unifying theory that really wants to see all quantities of fundamental objects as being close to «one» in the Planck units. And if some quantity is much smaller than the natural Planck unit, e.g. if the electron is much lighter than the Planck mass, it’s due to some cancellations that are known to occur almost everywhere in string theory.
But the fundamental parts of the explanations that matter – in this case, I mean the «positive-mass» part of the electron’s gyroscope – universally work in the regime where «everything is of order one in some units». Whenever dimensional analysis is any useful in string theory, except for telling you that everything is comparable to the Planck/string scale, it’s always in situations where some leading Planck-scale natural contributions «mostly cancel». Quantum field theorists like to think that any precise cancellation is «unnatural» but string theory offers us tons of totally sensible, justifiable, provable cancellations like that.
The cancellations resulting from supersymmetry represent a well-known example but string theory really does imply similar cancellations even in the absence of SUSY (or cancellations that don’t seem to be consequences of SUSY), too.